Convergence of a Series 

1.Sequence(Series)- 

Let S be any non-empty set.A function whose domain is the set N of natural numbers and whose range is a subset of S, is called a sequence in the set S.

 In other words a sequence in a set S is a rule which assigns to each natural number a unique element of S.
A sequence whose range is a subset of R is called a real sequence or a sequence of real numbers.
In this question we will solve and study only real sequences. therefore the term sequence will be used to denote a real sequence.
If s is a sequence,then the image ss(n) of N is usually denoted by s(n). It is customary to denote the sequence s by the symbol <s(n)>.The image s(n) of n is called the nth term of the sequence.

convergence of a series 

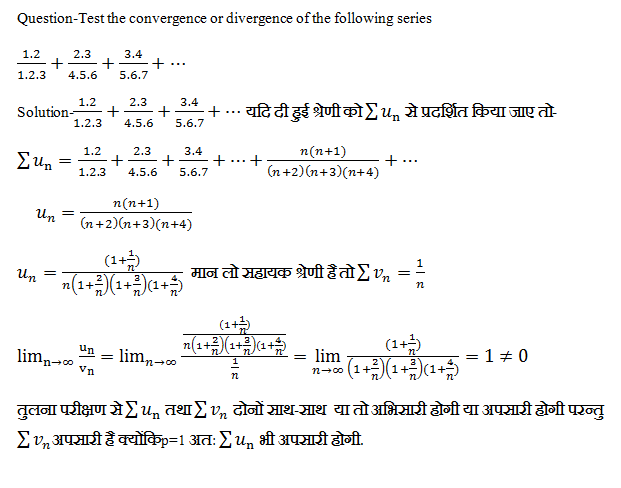2.A sequence(series) can be described in several different ways-

(1.)Listing in order the first few elements of a sequence,till the rule for writing down different elements becomes clear.For example,<1,8,27,64,...>is the sequence.
(2.)Defining a sequence by a formula for its nth term.For example,the sequence <1,8,27,64,....> can also be written as <1,8,27,.....,n^3,.....>